Space-time Singularities

General relativity, Einstein's theory of space, time, and gravity, allows for the existence of singularities. On this nearly all agree. However, when it comes to the question of how, precisely, singularities are to be defined, there is widespread disagreement. Singularties in some way signal a breakdown of the geometry itself, but this presents an obvious difficulty in referring to a singulary as a “thing” that resides at some location in spacetime: without a well-behaved geomtry, there can be no “location.” For this reason, some philosopers and physicists have suggested that we should not speak of “singularities” at all, but rather of “singular spacetimes.” In this entry, we shall generally treat these two formulations as being equivalent, but we will highlight the distinction when it becomes significant.

Singularities are often conceived of metaphorically as akin to a tear in the fabric of spacetime. The most common attempts to define singularities center on one of two core ideas that this image readily suggests.

The first is that a spacetime has a singularity just in case it contains an incomplete path, one that cannot be continued indefinitely, but draws up short, as it were, with no possibility of extension. (“Where is the path supposed to go after it runs into the tear? Where did it come from when it emerged from the tear?”). The second is that a spacetime is singular just in case there are points “missing from it.” (“Where are the spacetime points that used to be or should be where the tear is?”) Another common thought, often adverted to in discussion of the two primary notions, is that singular structure, whether in the form of missing points or incomplete paths, must be related to pathological behavior of some sort on the part of the singular spacetime's curvature, that is, the fundamental deformation of spacetime that manifests itself as “the gravitational field.” For example, some measure of the intensity of the curvature (“the strength of the gravitational field”) may increase without bound as one traverses the incomplete path. Each of these three ideas will be considered in turn below.

There is likewise considerable disagreement over the significance of singularties. Many eminent physicists believe that general relativity's prediction of singular structure signals a serious deficiency in the theory; singularities are an indication that the description offered by general relativity is breaking down. Others believe that singularities represent an exciting new horizon for physicists to aim for and explore in cosmology, holding out the promise of physical phenomena differing so radically from any that we have yet experienced as to ensure, in our attempt to observe, quantify and understand them, a profound advance in our comprehension of the physical world.

1 Path Incompleteness

While there are competing definitions of spacetime singularities, the most central, and widely accepted, criterion rests on the possibility that some spacetimes contain incomplete paths. Indeed, the rival definitions (in terms of missing points or curvature pathology) still make use of the notion of path incompleteness.

(The reader unfamiliar with general relativity may find it helpful to review the Hole Argument entry's Beginner's Guide to Modern Spacetime Theories, which presents a brief and accessible introduction to the concepts of a spacetime manifold, a metric, and a worldline.)

A path in spacetime is a continuous chain of events through space and time. If I snap my fingers continually, without pause, then the collection of snaps forms a path. The paths used in the most important singularity theorems represent possible trajectories of particles and observers. Such paths are known as “world-lines”; they consist of the events occupied by an object throughout its lifetime. That the paths be incomplete and inextendible means, roughly speaking, that, after a finite amount of time, a particle or observer following that path would “run out of world,” as it were—it would hurtle into the tear in the fabric of spacetime and vanish. Alternatively, a particle or observer could leap out of the tear to follow such a path. While there is no logical or physical contradiction in any of this, it appears on the face of it physically suspect for an observer or a particle to be allowed to pop in or out of existence right in the middle of spacetime, so to speak—if that does not suffice for concluding that the spacetime is “singular,” it is difficult to imagine what else would. At the same time, the ground-breaking work predicting the existence of such pathological paths produced no consensus on what ought to count as a necessary condition for singular structure according to this criterion, and thus no consensus on a fixed definition for it.

In this context, an incomplete path in spacetime is one that is both inextendible and of finite proper length, which means that any particle or observer traversing the path would experience only a finite interval of existence that in principle cannot be continued any longer. However, for this criterion to do the work we want it to, we'll need to limit the class of spacetimes under discussion. Specifically, we shall be concerned with spacetimes that are maximally extended (or just maximal). In effect, this condition says that one's representation of spacetime is “as big as it possibly can be”—there is, from the mathematical point of view, no way to treat the spacetime as being a proper subset of a larger, more extensive spacetime.

If there is an incomplete path in a spacetime, goes the thinking behind the requirement, then perhaps the path is incomplete only because one has not made one's model of spacetime big enough. If one were to extend the spacetime manifold maximally, then perhaps the previously incomplete path could be extended into the new portions of the larger spacetime, indicating that no physical pathology underlay the incompleteness of the path. The inadequacy would merely reside in the incomplete physical model we had been using to represent spacetime.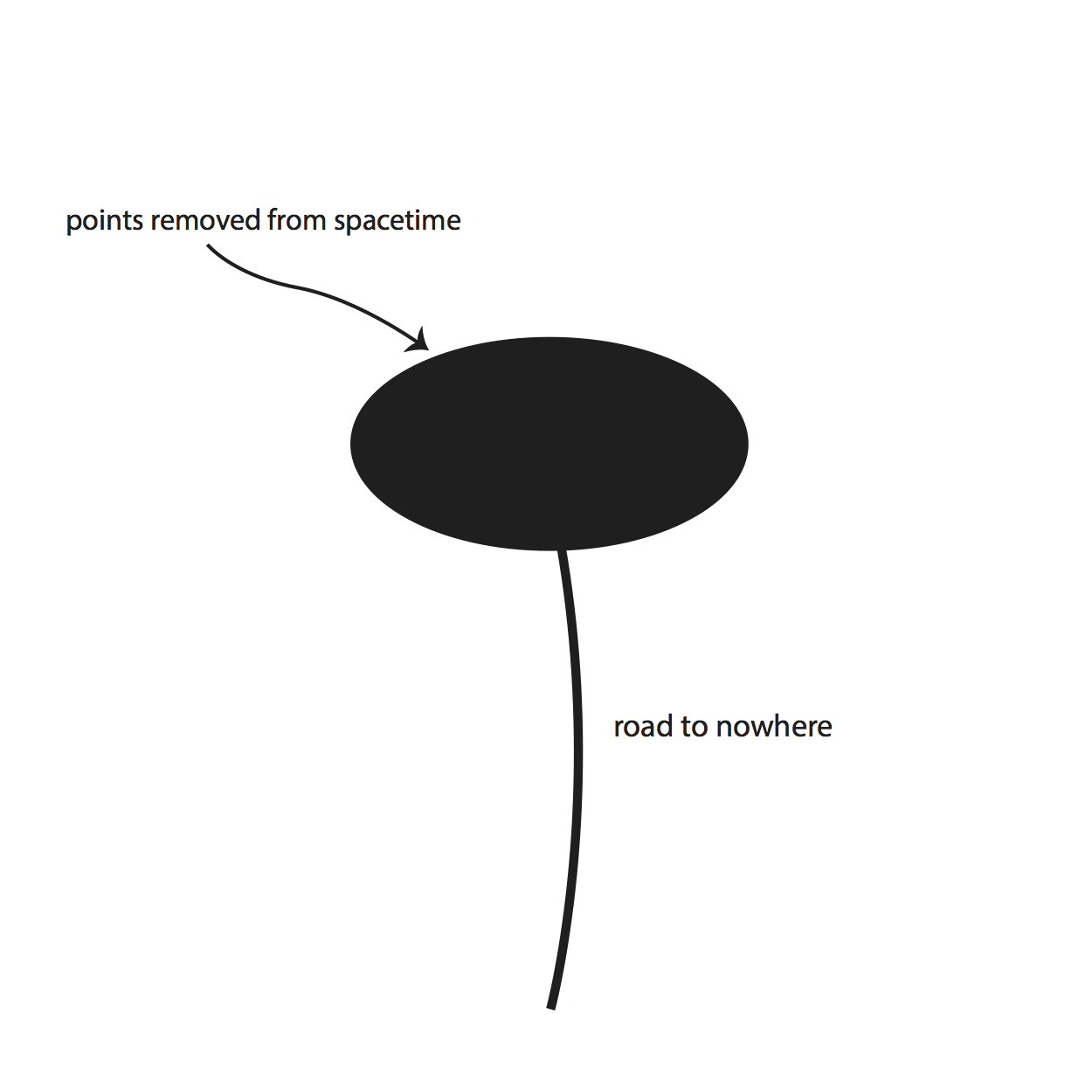

An example of a non-maximally extended spacetime can be easily had, along with a sense of why they intuitively seem in some way or other deficient. For the moment, imagine spacetime is only two-dimensional, and flat. Now, excise from somewhere on the plane a closed set shaped like Ingrid Bergman. Any path that had passed through one of the points in the removed set is now incomplete.

In this case, the maximal extension of the resulting spacetime is obvious, and does indeed fix the problem of all such incomplete paths: re-incorporate the previously excised set. The seemingly artificial and contrived nature of such examples, along with the ease of rectifying them, seems to militate in favor of requiring spacetimes to be maximal.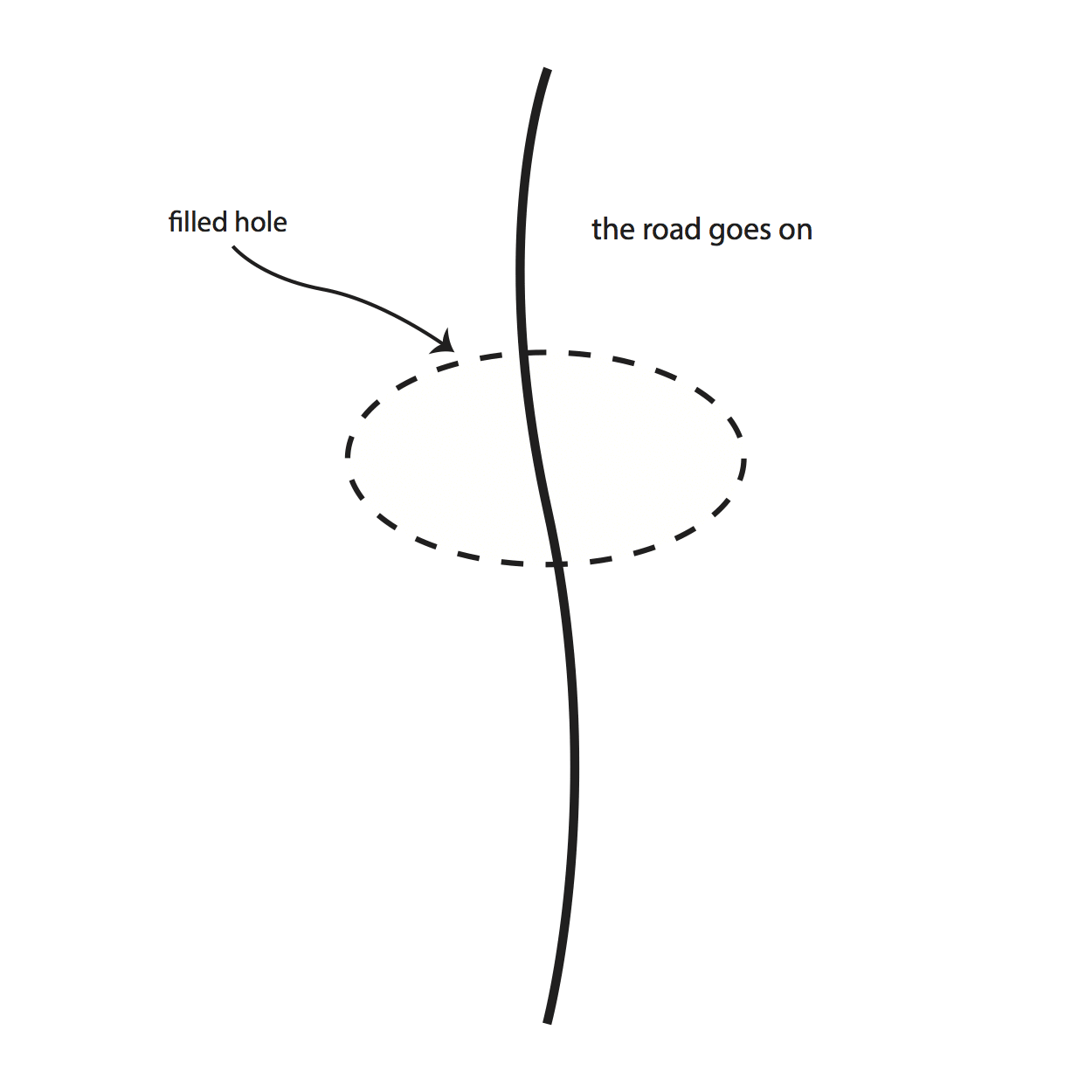

Once we've established that we're interested in maximal spacetimes, the next issue is what sort of path incompleteness is relevant for singularities. Here we find a good deal of controversy. Criteria of incompleteness typically look at how some parameter naturally associated with the path (such as its proper length) grows. One generally also places further restrictions on the paths that are worth considering (for example, one rules out paths that could only be taken by particles undergoing unbounded acceleration in a finite period of time). A spacetime is said to be singular if it possesses a path such that the specified parameter associated with that path cannot increase without bound as one traverses the entirety of the maximally extended path. The idea is that the parameter at issue will serve as a marker for something like the time experienced by a particle or observer, and so, if the value of that parameter remains finite along the whole path then we've run out of path in a finite amout of time, as it were. We've hit and “edge” or a “tear” in spacetime.

For a path that is everywhere timelike (i.e., that does not involves speeds at or above that of light), it is natural to take as the parameter the proper time a particle or observer would experience along the path, that is, the time measured along the path by a natural clock, such as one based on the natural vibrational frequency of an atom. (There are also fairly natural choices that one can make for spacelike paths (i.e., those that consist of points at a single “time”) and null paths (those followed by light signals). However, because the spacelike and null cases add yet another level of difficulty, we shall not discuss them here.) The physical interpretation of this sort of incompleteness for timelike paths is more or less straightforward: a timelike path incomplete with respect to proper time in the future direction would represent the possible trajectory of a massive body that would, say, never age beyond a certain point in its existence (an analogous statement can be made, mutatis mutandis, if the path were incomplete in the past direction).

We cannot, however, simply stipulate that a maximal spacetime is singular just in case it contains paths of finite proper length that cannot be extended. Such a criterion would imply that even the flat spacetime described by special relativity is singular, which is surely unacceptable. This would follow because, even in flat spacetime, there are timelike paths with unbounded acceleration which have only a finite proper length (proper time, in this case) and are also inextendible.

The most obvious option is to define a spacetime as singular if and only if it contains incomplete, inextendible timelike geodesics, i.e., paths representing the trajectories of inertial observers, those in free-fall experiencing no acceleration “other than that due to gravity.” However, this criterion seems too permissive, in that it would count as non-singular some spacetimes whose geometry seems quite pathological. For example, Geroch (1968) demonstrates that a spacetime can be geodesically complete and yet possess an incomplete timelike path of bounded total acceleration—that is to say, an inextendible path in spacetime traversable by a rocket with a finite amount of fuel, along which an observer could experience only a finite amount of proper time. Surely the intrepid astronaut in such a rocket, who would never age beyond a certain point but who also would never necessarily die or cease to exist, would have just cause to complain that something was singular about this spacetime.
We therefore want a definition that is not restricted to geodesics when deciding whether a spacetime is singular. However, we need some way of overcoming the fact that non-singular spacetimes include inextendible paths of finite proper length. The most widely accepted solution to this problem makes use of a slightly different (and slightly technical) notion of length, known as “generalized affine length.”^[1] Unlike proper length, this generalized affine length depends on some arbitrary choices (roughly speaking, the length will vary depending on the coordinates one chooses). However, if the length is infinite for one such choice, it will be infinite for all other choices. Thus the question of whether a path has a finite or infinite generalized affine length is a perfectly well-defined question, and that is all we'll need.

The definition that has won the most widespread acceptance — leading Earman (1995, p. 36) to dub this the semiofficial definition of singularities — is the following:

A maximal spacetime is singular if and only if it contains an inextendible path of finite generalized affine length.

To say that a spacetime is singular then is to say that there is at least one maximally extended path that has a bounded (generalized affine) length. To put it another way, a spacetime is nonsingular when it is complete in the sense that the only reason any given path might not be extendible is that it's already infinitely long (in this technical sense).

The chief problem facing this definition of singularities is that the physical significance of generalized affine length is opaque, and thus it is unclear what the relevance of singularities, defined in this way, might be. It does nothing, for example, to clarify the physical status of the spacetime described by Geroch; it seems as though the new criterion does nothing more than sweep the troubling aspects of such examples under the rug. It does not explain why we ought not take such prima facie puzzling and troubling examples as physically pathological; it merely declares by fiat that they are not.

So where does this leave us? The consensus seems to be that, while it is easy in specific examples to conclude that incomplete paths of various sorts represent singular structure, no entirely satisfactory, strict definition of singular structure in their terms has yet been formulated. For a philosopher, the issues offer deep and rich veins for those contemplating, among other matters, the role of explanatory power in the determination of the adequacy of physical theories, the role of metaphysics and intuition, questions about the nature of the existence attributable to physical entities in spacetime and to spacetime itself, and the status of mathematical models of physical systems in the determination of our understanding of those systems as opposed to in the mere representation our knowledge of them.

2 Boundary Constructions

We have seen that one runs into difficulties if one tries to define singularities as “things” that have “locations,” and how some of those difficulties can be avoided by defining singular spacetimes in terms of incomplete paths. However, it would be desirable for many reasons to have a characterization of a spacetime singularity in general relativity as, in some sense or other, a spatiotemporal “place.” If one had a precise characterization of a singularity in terms of points that are missing from spacetime, one might then be able to analyze the structure of the spacetime “locally at the singularity,” instead of taking troublesome, perhaps ill-defined limits along incomplete paths. Many discussions of singular structure in relativistic spacetimes, therefore, are premised on the idea that a singularity represents a point or set of points that in some sense or other is “missing” from the spacetime manifold, that spacetime has a “hole” or “tear” in it that we could fill in or patch by the appendage of a boundary to it.

In trying to determine whether an ordinary web of cloth has a hole in it, for example, one would naturally rely on the fact that the web exists in space and time. In this case one can, so to speak, point to a hole in the cloth by specifying points of space at a particular moment of time not currently occupied by any of the cloth but which would, as it were, complete the cloth were they so occupied. When trying to conceive of a singular spacetime, however, one does not have the luxury of imagining it embedded in a larger space with respect to which one can say there are points missing from it. In any event, the demand that the spacetime be maximal rules out the possibility of embedding the spacetime manifold in any larger spacetime manifold of any ordinary sort. It would seem, then, that making precise the idea that a singularity is a marker of missing points ought to devolve upon some idea of intrinsic structural incompleteness in the spacetime manifold rather than extrinsic incompleteness with respect to an external structure.

Force of analogy suggests that one define a spacetime to have points missing from it if and only if it contains incomplete, inextendible paths, and then try to use these incomplete paths to construct in some fashion or other new, properly situated points for the spacetime, the addition of which will make the previously inextendible paths extendible. These constructed points would then be our candidate singularities. Missing points on this view would correspond to a boundary for a singular spacetime—actual points of an extended spacetime at which paths incomplete in the original spacetime would terminate. (We will, therefore, alternate between speaking of missing points and speaking of boundary points, with no difference of sense intended.) The goal then is to construct this extended space using the incomplete paths as one's guide.

Now, in trivial examples of spacetimes with missing points such as the one offered before, flat spacetime with a closed set in the shape of Ingrid Bergman excised from it, one does not need any technical machinery to add the missing points back in. One can do it by hand, as it were. Many spacetimes with incomplete paths, however, do not allow “missing points” to be attached in any obvious way by hand, as this example does. For this program to be viable, which is to say, in order to give substance to the idea that there really are points that in some sense ought to have been included in the spacetime in the first place, we require a physically natural completion procedure based on the incomplete paths that can be applied to incomplete paths in arbitrary spacetimes.

Several problems with this program make themselves felt immediately. Consider, for example, an instance of spacetime representing the final state of the complete gravitational collapse of a spherically symmetric body resulting in a black hole. (See §3 below for a description of black holes.) In this spacetime, any timelike path entering the black hole will necessarily be extendible for only a finite amount of proper time—it then “runs into the singularity” at the center of the black hole. In its usual presentation, however, there are no obvious points missing from the spacetime at all. It is, to all appearances, as complete as the Cartesian plane, excepting only for the existence of incomplete curves, no class of which indicates by itself a place in the manifold to add a point to it to make the paths in the class complete. Likewise, in our own spacetime every inextendible, past-directed timelike path is incomplete (and our spacetime is singular): they all “run into the Big Bang.” Insofar as there is no moment of time at which the Big Bang occurred (there is no moment of time at which time began, so to speak), there is no point to serve as the past endpoint of such a path.

The reaction to the problems faced by these boundary constructions is varied, to say the least, ranging from blithe acceptance of the pathology (Clarke 1993), to the attitude that there is no satisfying boundary construction currently available without ruling out the possibility of better ones in the future (Wald 1984), to not even mentioning the possibility of boundary constructions when discussing singular structure (Joshi 1993), to rejection of the need for such constructions at all (Geroch, Can-bin and Wald, 1982).

Nonetheless, many eminent physicists seem convinced that general relativity stands in need of such a construction, and have exerted extraordinary efforts in the service of trying to devise such constructions. This fact raises several fascinating philosophical problems. Though physicists offer as strong motivation the possibility of gaining the ability to analyze singular phenomena locally in a mathematically well-defined manner, they more often speak in terms that strongly suggest they suffer a metaphysical, even an ontological, itch that can be scratched only by the sharp point of a localizable, spatiotemporal entity serving as the locus of their theorizing. However, even were such a construction forthcoming, what sort of physical and theoretical status could accrue to these missing points? They would not be idealizations of a physical system in any ordinary sense of the term, insofar as they would not represent a simplified model of a system formed by ignoring various of its physical features, as, for example, one may idealize the modeling of a fluid by ignoring its viscosity. Neither would they seem necessarily to be only convenient mathematical fictions, as, for example, are the physically impossible dynamical evolutions of a system one integrates over in the variational derivation of the Euler-Lagrange equations, for, as we have remarked, many physicists and philosophers seem eager to find such a construction for the purpose of bestowing substantive and clear ontic status on singular structure. What sorts of theoretical entities, then, could they be, and how could they serve in physical theory?

While the point of this project may seem at bottom identical to the path incompleteness account discussed in §1.1, insofar as singular structure will be defined by the presence of incomplete, inextendible paths, there is a crucial semantic and logical difference between the two. Here, the existence of the incomplete path is not taken itself to constitute the singular structure, but rather serves only as a marker for the presence of singular structure in the sense of missing points: the incomplete path is incomplete because it “runs into a hole” in the spacetime that, were it filled, would allow the path to be continued; this hole is the singular structure, and the points constructed to fill it compose its locus.

Currently, however, there seems to be even less consensus on how (and whether) one should define singular structure in terms of missing points than there is regarding definitions in terms of path incompleteness. Moreover, this project also faces even more technical and philosophical problems. For these reasons, path incompleteness is generally considered the default definition of singularities.

3 Curvature Pathology

While path incompleteness seems to capture an important aspect of the intuitive picture of singular structure, it completely ignores another seemingly integral aspect of it: curvature pathology. If there are incomplete paths in a spacetime, it seems that there should be a reason that the path cannot go farther. The most obvious candidate explanation of this sort is something going wrong with the dynamical structure of the spacetime, which is to say, with the curvature of the spacetime. This suggestion is bolstered by the fact that local measures of curvature do in fact blow up as one approaches the singularity of a standard black hole or the big bang singularity. However, there is one problem with this line of thought: no species of curvature pathology we know how to define is either necessary or sufficient for the existence of incomplete paths. (For a discussion of defining singularities in terms of curvature pathologies, see Curiel 1998.)

To make the notion of curvature pathology more precise, we will use the manifestly physical idea of tidal force. Tidal force is generated by the differential in intensity of the gravitational field, so to speak, at neighboring points of spacetime. For example, when you stand, your head is farther from the center of the Earth than your feet, so it feels a (practically negligible) smaller pull downward than your feet. (For a diagram illustrating the nature of tidal forces, see Figure 9 of the entry on Inertial Frames.) Tidal forces are a physical manifestation of spacetime curvature, and one gets direct observational access to curvature by measuring these forces. For our purposes, it is important that in regions of extreme curvature, tidal forces can grow without bound.

It is perhaps surprising that the state of motion of the observer as it traverses an incomplete path (e.g. whether the observer is accelerating or spinning) can be decisive in determining the physical response of an object to the curvature pathology. Whether the object is spinning on its axis or not, for example, or accelerating slightly in the direction of motion, may determine whether the object gets crushed to zero volume along such a path or whether it survives (roughly) intact all the way along it, as in examples offered by Ellis and Schmidt (1977). The effect of the observer's state of motion on his or her experience of tidal forces can be even more pronounced than this. There are examples of spacetimes in which an observer cruising along a certain kind of path would experience unbounded tidal forces and so be torn apart, while another observer, in a certain technical sense approaching the same limiting point as the first observer, accelerating and decelerating in just the proper way, would experience a perfectly well-behaved tidal force, though she would approach as near as one likes to the other fellow who is in the midst of being ripped to shreds.

Things can get stranger still. There are examples of incomplete geodesics contained entirely within a well-defined area of a spacetime, each having as its limiting point an honest-to-goodness point of spacetime, such that an observer freely falling along such a path would be torn apart by unbounded tidal forces; it can easily be arranged in such cases, however, that a separate observer, who actually travels through the limiting point, will experience perfectly well-behaved tidal forces. Here we have an example of an observer being ripped apart by unbounded tidal forces right in the middle of spacetime, as it were, while other observers cruising peacefully by could reach out to touch him or her in solace during the final throes of agony. This example also provides a nice illustration of the inevitable difficulties attendant on attempts to localize singular structure.

It would seem, then, that curvature pathology as standardly quantified is not in any physical sense a well-defined property of a region of spacetime simpliciter. When we consider the curvature of four-dimensional spacetime, the motion of the device that we use to probe a region (as well as the nature of the device) becomes crucially important for the question of whether pathological behavior manifests itself. This fact raises questions about the nature of quantitative measures of properties of entities in general relativity, and what ought to count as observable, in the sense of reflecting the underlying physical structure of spacetime. Because apparently pathological phenomena may occur or not depending on the types of measurements one is performing, it does not seem that this pathology reflects anything about the state of spacetime itself, or at least not in any localizable way.

What then may it reflect, if anything? Much work remains to be done by both physicists and by philosophers in this area, the determination of the nature of physical quantities in general relativity and what ought to count as an observable with intrinsic physical significance. See Bergmann (1977), Bergmann and Komar (1962), Bertotti (1962), Coleman and Korté (1992), and Rovelli (1991, 2001, 2002a, 2002b) for discussion of many different topics in this area, approached from several different perspectives.

[Courtesy: stanford]